Physical Significance:
The physical significance of Maxwell's equations obtained from integral form are given below:
Maxwell's First Equation:
1. The total electric displacement through the surface enclosing a volume is equal to the total charge within the volume.
2. It represents Gauss Law.
3. This law is independent of time.
Charge acts as source or sink for the lines of electric force.
Maxwell's Second Equation:
1. The total magnetic flux emitting through any closed surface is zero. An isolated magnet do not exist monopoles.
2. There is no source or sink for lines of magnetic force.
3. This is time independent equation.
Maxwell's Third Equation:
1. The electromotive force around the closed path is equal to the time derivative of the magnetic displacement through any surface bounded by the path.
2. This gives relation between electric field $E$ and magnetic induction $B$.
3. This expression is time varying i.e. $E$ is generated by time variation of $B$.
4.This gives relation in variation of E with the time variation of $B$ or $H$.
5. This is a mathematical form of Faraday's law of electromagnetic induction or Lenz's
Law.
Maxwell's Fourth Equation:
1. The magneto-motive force around the closed path is equal to the conduction current
plus displacement current through any surface bounded by the path.
2. This is time dependent wave equation.
3 This is a mathematical form of Ampere circuital law.
4. Magnetic induction $B$ can be generated from $J$ and time variation of magnetic displacement $D$.
5. This relates the space variation of $B$ with time variation of $D$.
Circuit containing Inductor and Capacitor in Series (L-C Series Circuit )
Mathematical Analysis of L-C Series Circuit :
Let us consider, a circuit containing inductor $L$ capacitor $C$ and these are connected in series. If an alternating voltage source is applied across it then the resultant voltage of the L-C circuit
$V=V_{L} - V_{C} \qquad(1)$
We know that:
$V_{L} = iX_{L}$
$V_{C} = iX_{C}$
So from equation $(1)$
$V= iX_{L} - iX_{C} $
$V=i \left(X_{L} - X_{C} \right) $
$\frac{V}{i}=\left(X_{L} - X_{C} \right) $
$Z=\left(X_{L} - X_{C} \right) \qquad(2)$
Where
$Z \rightarrow$ Impedance of L-C circuit.
$X_{L} \rightarrow$ Inductive Reactance which has value $\omega L$
$X_{C} \rightarrow$ Capacitive Reactance which has value $\frac{1}{\omega C}$
So from equation $(2)$, we get
$Z=\left( \omega L - \frac{1}{\omega C} \right) \qquad(3)$
The phase of resultant voltage:
The phase of resultant voltage from current is $90^{\circ}$ as shown in the figure above.
The Impedance and Phase at Resonance Condition:($X_{L} = X_{C}$):
At resonance $X_{L} = X_{C} \qquad(5)$
$\omega L = \frac{1}{\omega C}$
$\omega^{2} = \frac{1}{L C}$
$\omega = \sqrt{\frac{1}{L C}}$
$2 \pi f = \sqrt{\frac{1}{L C}}$
$ f = \frac{1}{2 \pi}\sqrt{\frac{1}{L C}}$
Where $f \rightarrow$ Natural frequency of the circuit.
1.) The Impedance of the circuit at resonance condition:
Substitute the resonance condition i.e. $X_{L} = X_{C}$ in equation $(2)$ then the impedance of the L-C Circuit
$Z=0$
The impedance of the L-C circuit at resonance condition is zero.
2.) The Phase of resultant voltage at resonance condition:
There is not any change in the phase of resultant voltage at resonance condition i.e. that will be the same $90^{\circ}$.
$V_{C} = iX_{C}$
$Z \rightarrow$ Impedance of L-C circuit.
$X_{L} \rightarrow$ Inductive Reactance which has value $\omega L$
$X_{C} \rightarrow$ Capacitive Reactance which has value $\frac{1}{\omega C}$
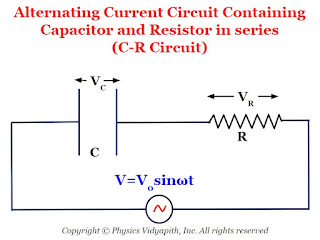
Circuit containing Capacitor and Resistor in Series (C-R Series Circuit )
Mathematical Analysis of C-R Series Circuit :
Let us consider, a circuit containing capacitor $C$ resistor $R$ and these are connected in series. If an alternating voltage source is applied across it then the resultant voltage of the C-R circuit
$V=\sqrt{ V_{C} ^{2} + V^{2}_{R}} \qquad(1)$
We know that:
$V_{R} = iR$
$V_{C} = iX_{C}$
So from equation $(1)$
$V=\sqrt{\left( iX_{C} \right)^{2} + \left(iR\right)^{2}} $
$V=i\sqrt{\left( X_{C} \right)^{2} + R^{2}} $
$\frac{V}{i}=\sqrt{\left( X_{C} \right)^{2} + R^{2}} $
$Z=\sqrt{\left( X_{C} \right)^{2} + R^{2}} \qquad(2)$
Where
$Z \rightarrow$ Impedance of C-R circuit.
$X_{C} \rightarrow$ Capacitive Reactance which has value $\frac{1}{\omega C}$
So from equation $(2)$, we get
$Z=\sqrt{\left( \frac{1}{\omega C} \right)^{2} + R^{2}} \qquad(3)$
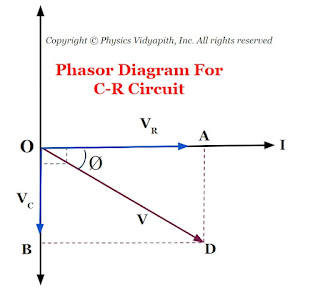
The phase of resultant voltage:
If the phase of resultant voltage from from current is $\phi$ then
$tan \phi = \frac{X_{C} }{R} \qquad(4)$
$tan \phi = \frac{\frac{1}{\omega C}}{R} $
$tan \phi = \frac{1}{\omega C R} $
$\phi = tan^{1} \left(\frac{1}{\omega C R}\right) $
$V_{C} = iX_{C}$
$Z \rightarrow$ Impedance of C-R circuit.
$X_{C} \rightarrow$ Capacitive Reactance which has value $\frac{1}{\omega C}$
Circuit containing Inductor and Resistor in Series (L-R Series Circuit )
Mathematical Analysis of L-R Series Circuit :
Let us consider, a circuit containing inductor $L$ resistor $R$ and these are connected in series. If an alternating voltage source is applied across it then the resultant voltage of the L-R circuit
$V=\sqrt{ V_{L} ^{2} + V^{2}_{R}} \qquad(1)$
We know that:
$V_{R} = iR$
$V_{L} = iX_{L}$
So from equation $(1)$
$V=\sqrt{\left( iX_{L} \right)^{2} + \left(iR\right)^{2}} $
$V=i\sqrt{\left( X_{L} \right)^{2} + R^{2}} $
$\frac{V}{i}=\sqrt{\left( X_{L} \right)^{2} + R^{2}} $
$Z=\sqrt{\left( X_{L} \right)^{2} + R^{2}} \qquad(2)$
Where
$Z \rightarrow$ Impedance of L-R circuit.
$X_{L} \rightarrow$ Inductive Reactance which has value $\omega L$
So from equation $(2)$, we get
$Z=\sqrt{\left( \omega L \right)^{2} + R^{2}} \qquad(3)$
The phase of resultant voltage:
If the phase of resultant voltage from from current is $\phi$ then
$tan \phi = \frac{X_{L} }{R} \qquad(4)$
$tan \phi = \frac{\omega L }{R} $
$\phi = tan^{1} \left(\frac{\omega L }{R}\right) $
$V_{L} = iX_{L}$
$Z \rightarrow$ Impedance of L-R circuit.
$X_{L} \rightarrow$ Inductive Reactance which has value $\omega L$
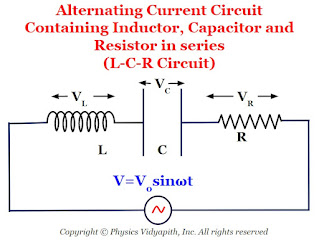
Circuit containing Inductor, Capacitor, and Resistor in Series (L-C-R Series Circuit )
Mathematical Analysis of L-C-R Series Circuit :
Let us consider, a circuit containing inductor $L$, capacitor $C$, and resistor $R$ and these are connected in series. If an alternating voltage source is applied across it then the resultant voltage of the L-C-R circuit
$V=\sqrt{\left( V_{L} -V_{C} \right)^{2} + V^{2}_{R}} \qquad(1)$
We know that:
$V_{R} = iR$
$V_{L} = iX_{L}$
$V_{C} = iX_{C}$
So from equation $(1)$
$V=\sqrt{\left( iX_{L} - iX_{C} \right)^{2} + (iR)^{2}} $
$V=i\sqrt{\left( X_{L} - X_{C} \right)^{2} + R^{2}} $
$\frac{V}{i}=\sqrt{\left( X_{L} - X_{C} \right)^{2} + R^{2}} $
$Z=\sqrt{\left( X_{L} - X_{C} \right)^{2} + R^{2}} \qquad(2)$
Where
$Z \rightarrow$ Impedance of L-C-R circuit.
$X_{L} \rightarrow$ Inductive Reactance which has value $\omega L$
$X_{C} \rightarrow$ Inductive Reactance which has value $\frac{1}{\omega C}$
So from equation $(2)$, we get
$Z=\sqrt{\left( \omega L - \frac{1}{\omega C} \right)^{2} + R^{2}} \qquad(3)$
The phase of resultant voltage:
If the phase of resultant voltage from from current is $\phi$ then
$tan \phi = \frac{X_{L} - X_{C}}{R} \qquad(4)$
$tan \phi = \frac{\omega L - \frac{1}{\omega C}}{R} $
$ \phi = tan^{-1} \left( \frac{\omega L - \frac{1}{\omega C}}{R} \right) $
The Impedance and Phase at Resonance Condition:($X_{L} = X_{C}$):
At resonance $X_{L} = X_{C} \qquad(5)$
$\omega L = \frac{1}{\omega C}$
$\omega^{2} = \frac{1}{L C}$
$\omega = \sqrt{\frac{1}{L C}}$
$2 \pi f = \sqrt{\frac{1}{L C}}$
$ f = \frac{1}{2 \pi}\sqrt{\frac{1}{L C}}$
Where $f \rightarrow$ Natural frequency of the circuit
1.) The Impedance of the circuit at resonance condition:
Substitute the resonance condition i.e. $X_{L} = X_{C}$ in equation $(2)$ then the impedance of the L-C-R Circuit
$Z=R$
The impedance of the L-C-R circuit at resonance condition is equal to the resistance of the resistor applied in a circuit.
2.) The Phase of resultant voltage at resonance condition:
$tan\phi =0$
$tan\phi = tan 0^{\circ}$
$\phi=0^{\circ}$
The phase of resultant voltage at resonance condition is zero. i.e. the direction of resultant voltage in the direction of current in the circuit.
Note: There are following cases arise in the L-C-R circuit at resonance condition
Case -1:
If $X_{L} \gt X_{C}$, the $tan \phi$ is positive, i.e. $\phi$ is positive. In this case, the voltage leads to the current. Therefore, the circuit is more inductive rather than capacitive or resistive.
Case -2:
If $X_{L} \lt X_{C}$, the $tan \phi$ is negative, i.e. $\phi$ is negative. In this case, the voltage lags behind the current. Therefore, the circuit has a more capacitance-dominated circuit.
Case -3:
If $X_{L} = X_{C}$, the $tan \phi$ is zero, i.e. $\phi$ is negative. In this case, the voltage and the current are in phase. Therefore, the circuit is purely resistive.
$V_{L} = iX_{L}$
$V_{C} = iX_{C}$
$Z \rightarrow$ Impedance of L-C-R circuit.
$X_{L} \rightarrow$ Inductive Reactance which has value $\omega L$
$X_{C} \rightarrow$ Inductive Reactance which has value $\frac{1}{\omega C}$
Popular Posts
-
Angle of Acceptance → "If incident angle of light on the core for which the incident angle on the core-cladding interface equals t...
-
Let $S$ be a point monochromatic source of light of wavelength $\lambda$ placed at the focus of collimating lens $L_{1}$. The light beam is ...
-
Maxwell's Equations: Maxwell's equation of the electromagnetic wave is a collection of four equations i.e. Gauss's law of elec...
-
Derivation of interference of light due to a wedge-shaped thin film: Interference of light due to wedge-shaped thin film The wedge...
-
Let a plane wavefront be incident normally on slit $S_{1}$ and $S_{2}$ of equal $e$ and separated by an opaque distance $d$.The diffracted l...
Study-Material
Categories
Alternating Current Circuits
(10)
Atomic and Molecular Physics
(4)
Biomedical
(1)
Capacitors
(6)
Classical Mechanics
(12)
Current carrying loop in magnetic field
(5)
Current Electricity
(10)
Dielectric Materials
(1)
Electromagnetic Induction
(3)
Electromagnetic Wave Theory
(23)
Electrostatic
(22)
Energy Science and Engineering
(2)
Error and Measurement
(2)
Gravitation
(10)
Heat and Thermodynamics
(3)
Kinematics Theory Of Gases
(2)
Laser System & Application
(15)
Magnetic Effect of Current
(9)
Magnetic Substances
(3)
Mechanical Properties of Fluids
(4)
Nanoscience & Nanotechnology
(4)
Nuclear Physics
(7)
Numerical Problems and Solutions
(1)
Optical Fibre
(5)
Optics
(25)
Photoelectric Effect
(3)
Quantum Mechanics
(33)
Relativity
(8)
Semiconductors
(2)
Superconductors
(1)
Topic wise MCQ
(9)
Units and Dimensions
(1)
Waves
(5)