Gauss's Law:
Gauss's law for electric flux is given by
Carl Friedrich Gauss in 1813. He extended the work of
Joseph-Louis Lagrange. This formula was first formulated in 1713 by Lagrange. Gauss's law stated that:
The electric flux passing normal through any closed hypothetical surface is always equal to the $\frac{1}{\epsilon_{0}}$ times of the total charge enclosed within that closed surface. This closed hypothetical surface is known as Gaussian surface.
Let us consider that a $+q$ coulomb charge is enclosed within the Gaussian's surface. Then according to Gauss's Law, the electric flux will be:
|
$\phi _{E}= \frac{q}{\epsilon_{0}}$
|
The electric flux of the electric field →
$\phi_{E}=\oint \overrightarrow{E}\cdot\overrightarrow{dA}$
Substitute this value of electric flux $\phi_{E}$ in the above formula so we get →
|
$\oint
\overrightarrow{E}\cdot\overrightarrow{dA}=\frac{q}{\epsilon_{0}}$
|
Where $\epsilon_{0}$ → Permittivity of the free space
The above formula of Gauss's law is applicable only under the following two conditions:
- The electric field at every point on the surface is either perpendicular or tangential.
- The magnitude of the electric field at every point where it is perpendicular to the surface has a constant value.
Derivation of Gauss's law from Coulomb's law:
- When the charge is within the surface
- When the charge is outside the surface
1. When the charge is within the surface:
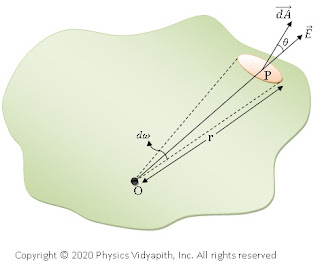
Let a charge $+q$ is placed at point $O$ within a closed surface of irregular shape. Consider a point $P$ on the surface which is at a distance $r$ from the point $O$. Now take a small element or area $\overrightarrow{dA}$ around the point $P$. If $\theta$ is the angle between $\overrightarrow{E}$ and $\overrightarrow{dA}$ then electric flux through small element or area $\overrightarrow{dA}$
$d\phi_{E}=\overrightarrow{E}\cdot\overrightarrow{dA}$
$d\phi_{E}=E\:dA\:cos\theta \qquad\quad\quad (1)$
 |
| When charge is inside the surface |
According to Coulomb's law, the electric field intensity $E$ at point $P$.
$E=\frac{1}{4\pi\epsilon_{0}}\frac{q}{r^{2}}$
Now substitute the value of electric field intensity $E$ in equation $(1)$
$d\phi_{E}=\frac{q}{4\pi\epsilon_{0}}\frac{dA\:cos\theta}{r^{2}}$
but $\frac{dA\:cos\theta}{r^{2}}$ is the solid angle $d\omega$ subtended by $dA$ at point $O$. Hence the above equation can be written as
$d\phi_{E}=\frac{q}{4\pi\epsilon_{0}}d\omega$
So, The total flux $\phi_{E}$ over the entire surface can be found by integrating the above equation
$\oint d\phi_{E}= \frac{q}{4\pi\epsilon_{0}}\oint d\omega$
For entire surface solid angle $d\omega$ will be equal to $4\pi$ i.e. $d\omega=4\pi$
|
$\phi _{E}= \frac{q}{\epsilon_{0}}$
|
If the closed surface enclosed with several charges like $q_{1},q_{2},q_{3},.....-q_{1},-q_{2},-q_{3},.....$. Now each charge will contribute to the total electric flux $\phi_{E}$.
$\phi_{E}= \frac{1}{\epsilon_{0}}\left [ q_{1}+q_{2}+q_{3}...-q_{1}-q_{2}-q_{3}... \right ]$
Here $\quad q=q_{i}-q_{j}$
|
$\phi_{E}= \frac{1}{\epsilon_{0}}\sum_{i=1,j=1}^{n}(q_{i}-q_{j})$
|
|
$\phi_{E}= \frac{1}{\epsilon_{0}}\sum q$
|
Where $\sum q$ → Algebraic Sum of all the charges
2. When the charge is outside the surface:
Let a point charge $+q$ be situated at point $O$ outside the closed surface. Now a cone of solid angle $d\omega$ from point $O$ cuts the surface area $dA_{1}$, $dA_{2}$, $dA_{3}$, $dA_{4}$ at pont $P$, $Q$, $R$ and $S$ respectively. The electric flux for an outward normal is positive while for inward normal is negative so
The electric flux at point $P$ through area
$d\phi_{1}$= $-\left (\frac{q}{4\pi \epsilon_{0}} \right )d\omega$
The electric flux at point $Q$ through area
$d\phi_{2}$= $+\left (\frac{q}{4\pi \epsilon_{0}} \right )d\omega$
The electric flux at point $R$ through area
$d\phi_{3}$= $-\left (\frac{q}{4\pi \epsilon_{0}} \right )d\omega$
The electric flux at point $S$ through area
$d\phi_{4}$= $+\left (\frac{q}{4\pi \epsilon_{0}} \right )d\omega$
 |
| Charge is outside the surface |
The Total electric flux will be sum of all the electric flux passing through areas of surface →
$\phi_{E}=d\phi_{1}+d\phi_{2}+d\phi_{3}+d\phi_{4}$
$\phi_{E}=-\left ( \frac{q}{4\pi \epsilon_{0}} \right )d\omega+\left ( \frac{q}{4\pi \epsilon_{0}} \right )d\omega \\ \qquad \: -\left ( \frac{q}{4\pi
\epsilon_{0}} \right )d\omega+\left ( \frac{q}{4\pi \epsilon_{0}} \right )d\omega$
The above equation is true for all cones from point $O$ through any surface, however irregular it may be-
The total electric flux over the entire surface due to an external charge is zero.
This verifies Gauss's law.
Application of Gauss's law:
There are following some important application given below:
- Electric field intensity due to a point charge
- Electric field intensity due to uniformly charged spherical Shell (for Thin and Thick)
- Electric field intensity due to a uniformly charged solid sphere (Conducting and Non-conducting)
- Electric field intensity due to uniformly charged infinite plane sheet (for Thin and Thick)
- Electric field intensity due to uniformly charged parallel sheet
- Electric field intensity due to charged infinite length wire
