Statement of Principle of Continuity:
When an ideal liquid (i.e. incompressible and non-viscous liquid ) flows in streamlined motion through a tube of non-uniform cross-section, then the product of the velocity of flow and area of cross-section is always constant at every point in the tube.
Mathematical Analysis (Proof)
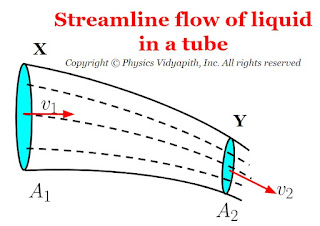
Let us consider, an ideal liquid (i.e. incompressible and non-viscous liquid ) flow in streamline motion through a tube $XY$ of the non-uniform cross-section.
Now Consider:
The Area of cross-section $X = A_{1}$
The Area of cross-section $Y = A_{2}$
The velocity per second (i.e. equal to distance) of fluid at cross-section $X = v_{1}$
The velocity per second (i.e. equal to distance) of fluid at cross-section $Y = v_{2}$
The volume of liquid entering at the cross-section $X$ in $1$ second is = $A_{1}v_{1}$
The mass of liquid entering at the cross-section $X$ in $1$ second is = $ \rho A_{1}v_{1}$
Similarly, the mass of the liquid coming out from the cross-section $Y$ in $1$ second is = $ \rho A_{2}v_{2}$
But the liquid which enters at cross-section $X$ must leave cross-section $Y$. Hence the masses are equal at both cross-section area, that is,
$\rho A_{1}v_{1} = \rho A_{2}v_{2} $
$ A_{1}v_{1} = A_{2}v_{2} $
$A v = constant$
Thus, the above equation shows that at every place in the tube, the product of the area of the cross-section of the tube and the velocity of the flow of the liquid is always the same. Therefore, the velocity of the liquid is smaller in the wider parts of the tube and larger in the narrow parts.
Combined Focal Length and Power of two thin lenses in contact
Derivation of the combined focal length and power of two thin lenses in contact:
Case (1): When both are convex lens-
a.) The combined focal length of two thin convex lenses in contact:
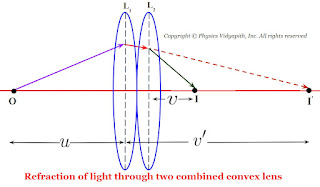
Let us consider that two convex lenses $L_{1}$ and $L_{2}$ are connected with transparent cement Canada Balsam. If the focal length of the lenses is $f_{1}$ and $f_{1}$ and an object $O$ is placed at distance $u$ from the first lens $L_{1}$ and its image $I'$ is formed at a distance $v'$ from the first lens $L_{1}$. Therefore from the equation of focal length for lens $L_{1}$
$\frac{1}{f_{1}} = \frac{1}{v'} - \frac{1}{u} \qquad(1)$
For the second lens, The image $I'$ works as a virtual object for the second lens $L_{2}$ which image $I$ is formed at a distance $v$ from the second lens $L_{2}$. Therefore from the equation of focal length for lens $L_{2}$
$\frac{1}{f_{2}} = \frac{1}{v} - \frac{1}{v'} \qquad(2)$
Now add the equation $(1)$ and equation $(2)$. then
$\frac{1}{f_{1}} + \frac{1}{f_{2}} = \frac{1}{v'} - \frac{1}{u} + \frac{1}{v} - \frac{1}{v'} $
$\frac{1}{f_{1}} + \frac{1}{f_{2}} = \frac{1}{v} - \frac{1}{u} $
Where $ \frac{1}{f} = \frac{1}{v} - \frac{1}{u} $
$\frac{1}{f_{1}} + \frac{1}{f_{2}} = \frac{1}{f} \qquad(3)$
$\frac{1}{f} = \frac{f_{1} + f_{2}}{f_{1}f_{2}} $
$f = \frac{f_{1}f_{2}}{f_{1} + f_{2}} $
This is the equation of the combined focal length of two thin convex lenses in contact.
b.)The combined power of two thin convex lenses in contact:
We know that the power of the lens equation
$P = \frac{1}{f}$
So from equation $(3)$
$P=P_{1} + P_{2}$
This is the equation of the combined power of two thin convex lenses in contact.
Similarly for another two cases $(2)$ and case $(3)$:
Case (2): When both are concave lens-
a.) The combined focal length of two thin concave lenses in contact:
For concave lenses the focal length for both lenses i.e. ($f_{1} \: and \: f_{2}$) will be negative. Therefore the combined focal length of the two thin concave lens
$ \frac{1}{f} = \frac{1}{- f_{1}} + \frac{1}{- f_{2}} $
$\frac{1}{f} =- \frac{\left(f_{1} + f_{2} \right)}{f_{1}f_{2}} $
$f = -\frac{f_{1}f_{2}}{f_{1} + f_{2}} $
b.) The combined power of two thin concave lenses in contact:
$P= - \left(P_{1} + P_{2}\right)$
Case (3): When one lens is convex and the second is concave-
a.) The combined focal length of two thin lenses for convex and concave:
If the focal length of convex lens is $f_{1}$ and for concave is $f_{2}$ then the combine focal length of lenses
$ \frac{1}{f} = \frac{1}{ f_{1}} + \frac{1}{- f_{2}} $
$\frac{1}{f} = \frac{\left(f_{1} - f_{2} \right)}{f_{1}f_{2}} $
$f = \frac{f_{1}f_{2}}{f_{1} - f_{2}} $
b.) The combined power of two thin lenses for convex and concave:
$P= \left(P_{1} - P_{2}\right)$
Refraction of light through a thin lens : Lens maker's formula
Derivation of refraction of light through a thin lens & Lens maker's formula:
Let us consider, A convex lens having thickness $t$ and radius of curvature of surfaces is $R_{1}$ and $R_{2}$. If an object $O$ is placed at distance $u$ from the first surface of the convex lens and its image $I'$ is formed at distance $v'$ from the first surface of the convex lens then refraction of light through the first spherical surface of the lens
$ \frac{\left( n_{2} - n_{1} \right)}{R_{1}} = \frac{n_{2}}{v'} - \frac{n_{1}}{u} \qquad(1) $
Now the Image $I'$ works as a virtual object for the second surface of the convex lens which image $I$ formed at distance $v$ from the second surface of the lens. So refraction of light through the second surface of the lens
$ \frac{\left( n_{1} - n_{2} \right)}{R_{2}} = \frac{n_{1}}{v} - \frac{n_{2}}{v' - t} $
Here $t$ is the thickness of the lens. If the lens is very thin then thickness will be $t=0$. Therefore above equation for second surface of the lens can be written as
$ \frac{\left( n_{1} - n_{2} \right)}{R_{2}} = \frac{n_{1}}{v} - \frac{n_{2}}{v'} \qquad(2) $
Now add the equation $(1)$ and equation $(2)$, So
$\frac{\left( n_{2} - n_{1} \right)}{R_{1}} + \frac{\left( n_{1} - n_{2} \right)}{R_{2}} = \frac{n_{2}}{v'} - \frac{n_{1}}{u} + \frac{n_{1}}{v} - \frac{n_{2}}{v'} $
$\frac{\left( n_{2} - n_{1} \right)}{R_{1}} + \frac{\left( n_{1} - n_{2} \right)}{R_{2}} = - \frac{n_{1}}{u} + \frac{n_{1}}{v} $
$\left( n_{2} - n_{1} \right) \left( \frac{1}{R_{1}} - \frac{1}{R_{2}} \right) =n_{1} \left( \frac{1}{v} - \frac{1}{u} \right) $
$n_{1} \left( \frac{1}{v} - \frac{1}{u} \right) = \left( n_{2} - n_{1} \right) \left( \frac{1}{R_{1}} - \frac{1}{R_{2}} \right) $
$ \left( \frac{1}{v} - \frac{1}{u} \right) = \frac{\left( n_{2} - n_{1} \right)}{n_{1}} \left( \frac{1}{R_{1}} - \frac{1}{R_{2}} \right) $
We know that the equation of the focal length of a lens
$\frac{1}{f} = \frac{1}{v} - \frac{1}{u}$
Where $f \rightarrow$ Focal length of convex lens. Now substitute the value of $f$ in the above equation
$ \frac{1}{f} = \frac{\left( n_{2} - n_{1} \right)}{n_{1}} \left( \frac{1}{R_{1}} - \frac{1}{R_{2}} \right)$
$ \frac{1}{f} = \left( \frac{n_{2}}{n_{1}} - 1 \right) \left( \frac{1}{R_{1}} - \frac{1}{R_{2}} \right)$
$ \frac{1}{f} = \left( n - 1 \right) \left( \frac{1}{R_{1}} - \frac{1}{R_{2}} \right) \qquad \left( \because \frac{n_{2}}{n_{1}}= n\right)$
The above equation represents the equation of refraction of light through a thin lens and lens maker's formula.
Refraction of light through the convex spherical surface
Derivation of refraction of light through the convex spherical surface:
Let us consider, a convex spherical surface which has radius of curvature $R$. If an object $O$ is placed at a distance $u$ from pole $P$ and its image $I$ is formed at distance $v$ from pole $P$ and the angle subtended by the object, image, and center of curvature is $\alpha$, $\beta$, and $\gamma$ then from figure
In $\Delta MOC$
$i= \gamma + \alpha \qquad(1)$
In $\Delta MIC$
$r = \gamma + \beta \qquad(2)$
According to Snell's Law:
$\frac{sin \: i}{sin \: r} = \frac{n_{2}}{n_{1}} \qquad(3)$
Here the aperture of the spherical surface is very small so point $M$ will be very close to point $P$ and angle $i$ and $r$ will be small. So
$sin \: i \approx i$
$sin \: r \approx r$
So equation $(3)$ can be written as
$\frac{ i}{ r} = \frac{n_{2}}{n_{1}} \qquad(4)$
Now subtitute the value of $i$ and $r$ from equation $(1)$ and equation $(2)$ in equation $(4)$
$\frac{ \left( \gamma + \alpha \right)}{\left( \gamma + \beta \right)} = \frac{n_{2}}{n_{1}} $
$ \left( \gamma + \alpha \right) n_{1} = \left( \gamma + \beta \right) n_{2} $
$ \gamma \: n_{1} + \alpha \: n_{1} = \gamma \: n_{2} + \beta \: n_{2} $
$ \gamma \: n_{1} - \gamma \: n_{2} = \beta \: n_{2} - \alpha \: n_{1} $
$ \left( n_{1} - n_{2} \right) \gamma = \beta \: n_{2} - \alpha \: n_{1} \qquad(5)$
Again here the aperture of the spherical surface is very small so point $N$ will be very close to point $P$. Therefore the angle $\alpha$, $\beta$, and $\gamma$ will be very small. Then in $\Delta MON$
$\alpha = tan \alpha = \frac {MN}{ON} \approx \frac{MN}{OP} = \frac{h}{-u}$
Similarly for angle $\beta$ and $\gamma$, Apply in $\Delta MIN$ and $\Delta MCN$
$\beta = tan \beta = \frac {MN}{IN} \approx \frac{MN}{IP} = \frac{h}{-v}$
$\gamma = tan \gamma = \frac {MN}{CN} \approx \frac{MN}{CP} = \frac{h}{R}$
Now subtitute the value of $\alpha$, $\beta$, and $\gamma$ from above in equation $(5)$
$ \left( \frac{h}{R} \right) \left( n_{1} - n_{2} \right) = \left( \frac{h}{-v} \right) n_{2} - \left( \frac{h}{-u} \right) n_{1} $
$ \frac{\left( n_{2} - n_{1} \right)}{R} = \frac{n_{2}}{v} - \frac{n_{1}}{u} $
This is the equation of refraction of light through a convex spherical surface.
Another form of the above equation:
$ n_{1} \frac{\left( \frac{n_{2}}{n_{1}} - 1 \right)}{R} = n_{1} \frac{\frac{n_{2}}{n_{1}}}{v} - \frac{1}{u} $
$ n_{1} \frac{\left( \frac{n_{2}}{n_{1}} - 1 \right)}{R} = n_{1} \frac{\left(\frac{n_{2}}{n_{1}}\right)}{v} - \frac{1}{u} $
$ \frac{\left( \frac{n_{2}}{n_{1}} - 1 \right)}{R} = \frac{\left(\frac{n_{2}}{n_{1}}\right)}{v} - \frac{1}{u} $
$ \frac{\left( n - 1 \right)}{R} = \frac{n}{v} - \frac{1}{u} \qquad \left( \because \frac{n_{2}}{n_{1}}= n\right) $
Where $n \rightarrow Refractive \: index \: of \: glass \: with \: respect \: to \: air$
This is another form of the equation of refraction of light through a convex spherical surface.
$sin \: r \approx r$
Refraction of light through the concave spherical surface
Derivation of refraction of light through the concave spherical surface:
Let us consider, a concave spherical surface of radius of curvature $R$. If an object $O$ is placed at a distance $u$ from pole $P$ and its image $I$ is formed at distance $v$ from pole $P$ and the angle subtended by the object, image, and center of curvature is $\alpha$, $\beta$, and $\gamma$ then from figure
In $\Delta MOC$
$\gamma= \alpha + i $
$i= \gamma - \alpha \qquad(1)$
In $\Delta MIC$
$\gamma= \beta + r $
$r = \gamma - \beta \qquad(2)$
According to Snell's Law:
$\frac{sin \: i}{sin \: r} = \frac{n_{2}}{n_{1}} \qquad(3)$
Here the aperture of the spherical surface is very small so point $M$ will be very close to point $P$ and angle $i$ and $r$ will be small. So
$sin \: i \approx i$
$sin \: r \approx r$
So equation $(3)$ can be written as
$\frac{ i}{ r} = \frac{n_{2}}{n_{1}} \qquad(4)$
Now subtitute the value of $i$ and $r$ from equation $(1)$ and equation $(2)$ in equation $(4)$
$\frac{ \left( \gamma - \alpha \right)}{\left( \gamma - \beta \right)} = \frac{n_{2}}{n_{1}} $
$ \left( \gamma - \alpha \right) n_{1} = \left( \gamma - \beta \right) n_{2} $
$ \gamma \: n_{1} - \alpha \: n_{1} = \gamma \: n_{2} - \beta \: n_{2} $
$ \gamma \: n_{1} - \gamma \: n_{2} = -\beta \: n_{2} + \alpha \: n_{1} $
$ \left( n_{1} - n_{2} \right) \gamma = -\beta \: n_{2} + \alpha \: n_{1} \qquad(5)$
Again here the aperture of the spherical surface is very small so point $N$ will be very close to point $P$. Therefore the angle $\alpha$, $\beta$, and $\gamma$ will be very small. Then in $\Delta MON$
$\alpha = tan \alpha = \frac {MN}{ON} \approx \frac{MN}{OP} = \frac{h}{-u}$
Similarly for angle $\beta$ and $\gamma$, Apply in $\Delta MIN$ and $\Delta MCN$
$\beta = tan \beta = \frac {MN}{IN} \approx \frac{MN}{IP} = \frac{h}{-v}$
$\gamma = tan \gamma = \frac {MN}{CN} \approx \frac{MN}{CP} = \frac{h}{-R}$
Now subtitute the value of $\alpha$, $\beta$, and $\gamma$ from above in equation $(5)$
$ \left( \frac{h}{-R} \right) \left( n_{1} - n_{2} \right) = - \left( \frac{h}{-v} \right) n_{2} + \left( \frac{h}{-u} \right) n_{1} $
$ \frac{\left( n_{2} - n_{1} \right)}{R} = \frac{n_{2}}{v} - \frac{n_{1}}{u} $
This is the equation of refraction of light through a concave spherical surface.
Another form of the above equation:
$ n_{1} \frac{\left( \frac{n_{2}}{n_{1}} - 1 \right)}{R} = n_{1} \frac{\frac{n_{2}}{n_{1}}}{v} - \frac{1}{u} $
$ n_{1} \frac{\left( \frac{n_{2}}{n_{1}} - 1 \right)}{R} = n_{1} \frac{\left(\frac{n_{2}}{n_{1}}\right)}{v} - \frac{1}{u} $
$ \frac{\left( \frac{n_{2}}{n_{1}} - 1 \right)}{R} = \frac{\left(\frac{n_{2}}{n_{1}}\right)}{v} - \frac{1}{u} $
$ \frac{\left( n - 1 \right)}{R} = \frac{n}{v} - \frac{1}{u} \qquad \left( \because \frac{n_{2}}{n_{1}}= n\right) $
Where $n \rightarrow Refractive \: index \: of \: glass \: with \\ respect \: to \: air$
This is another form of the equation of refraction of light through a concave spherical surface.
$sin \: r \approx r$
Popular Posts
-
Angle of Acceptance → "If incident angle of light on the core for which the incident angle on the core-cladding interface equals t...
-
Let $S$ be a point monochromatic source of light of wavelength $\lambda$ placed at the focus of collimating lens $L_{1}$. The light beam is ...
-
Maxwell's Equations: Maxwell's equation of the electromagnetic wave is a collection of four equations i.e. Gauss's law of elec...
-
Derivation of interference of light due to a wedge-shaped thin film: Interference of light due to wedge-shaped thin film The wedge...
-
Let a plane wavefront be incident normally on slit $S_{1}$ and $S_{2}$ of equal $e$ and separated by an opaque distance $d$.The diffracted l...
Study-Material
Categories
Alternating Current Circuits
(10)
Atomic and Molecular Physics
(4)
Biomedical
(1)
Capacitors
(6)
Classical Mechanics
(12)
Current carrying loop in magnetic field
(5)
Current Electricity
(10)
Dielectric Materials
(1)
Electromagnetic Induction
(3)
Electromagnetic Wave Theory
(23)
Electrostatic
(22)
Energy Science and Engineering
(2)
Error and Measurement
(2)
Gravitation
(10)
Heat and Thermodynamics
(3)
Kinematics Theory Of Gases
(2)
Laser System & Application
(15)
Magnetic Effect of Current
(9)
Magnetic Substances
(3)
Mechanical Properties of Fluids
(4)
Nanoscience & Nanotechnology
(4)
Nuclear Physics
(7)
Numerical Problems and Solutions
(1)
Optical Fibre
(5)
Optics
(25)
Photoelectric Effect
(3)
Quantum Mechanics
(33)
Relativity
(8)
Semiconductors
(2)
Superconductors
(1)
Topic wise MCQ
(9)
Units and Dimensions
(1)
Waves
(5)