Mechanism of Flow of Charge in Metals: Free Electron Gas Theory

Theory of Free Electron Gas Model → According to the electron gas theory

1. The free electrons are continuous in motion inside the metal. The motion of free electrons are random inside the metal.

2. When the free electrons are collisied to each other then the direction of electrons are changed.

3. Mean free Path: The length covered by free electrons, between the two successive collisions is called the "Mean Free Path".

4. Relaxation Time: The time taken between the two successive collisions of free electrons is called the Relaxation time. It is represented by $\tau$.

5. Drift velocity: When a potential is applied across the metal then these electrons do not move own velocity but it move with an average velocity in the opposite direction of the electric field. This average velocity is called the "Drift Velocity". The drift velocity of electrons depends upon the applied potential.

6. Mobility of Electrons: When a potential $V$ is applied across the metal then electrostatic force $F$ acts on the electrons i.e

$F=qE $

$F=NeE \qquad(1)$

Where
$N$ →The number of free electrons inside the metal
$E$ → The electric field due to the applied potential

When this electrostatic force is applied to the electrons then these electrons are accelerated with accelerated $a$ i.e

$F=ma \qquad(2)$

From equation $(1)$ and equation $(2)$ we can write

$ma=N\:e\:E$

$a=\frac{N}{m}eE$

$\frac{v_{d}}{\tau}=\frac{N}{m}eE \qquad \left( \because a=\frac{v_{d}}{\tau} \right)$

$v_{d}=\frac{Ne\tau}{m} E$

$v_{d}=\mu E$

Where $\mu=\frac{Ne\tau}{m}$. It is known as the mobility of electrons.

Read More

Derivation of Ohm's Law

Derivation→

Let us consider,

The length of the conductor = $l$
The cross-section area of the conductor = $A$
The potential difference across the conductor = $V$
The drift velocity of an electron in conductor = $v_{d}$

Now from the equation of the mobility of electron i.e.

$v_{d}= \mu E$

$v_{d}= \left( \frac{e\tau}{m} \right) E \qquad \left(\because \tau = \frac{e\tau}{m} \right)$

$v_{d}= \left( \frac{e\tau}{m} \right) \frac{V}{l}\qquad (1) \qquad \left(\because E = \frac{V}{l} \right)$

Now from the equation of drift velocity and electric current

$i=neAv_{d}$

Now substitute the value of $v_{d}$ from equation $(1)$ to above equation

$i=neA\left( \frac{e\tau}{m} \right) \frac{V}{l}$

$i=\left( \frac{ne^{2}A\tau}{ml} \right)V$

$\frac{V}{i}=\left( \frac{ml}{ne^{2}A\tau} \right)$

$\frac{V}{i}=R$

Where $R = \frac{ml}{ne^{2}A\tau} $ is known as electrical resistance of the conductor.

Thus

$V=iR$

This is Ohm's Law.

Read More

Relation between electric current and drift velocity

Derivation→ Let us consider

The length of the conductor = $l$

The cross-section area of the conductor = $A$

The total number of free electrons inside the conductor = $N$

The current flow in the conductor = $i$

The flow of Electron in Conductor

The Relaxation time between the two successive collisions =$\tau$

According to the law of current density

$J=\frac{i} {A} $

$J=\frac{q} {A \tau} \qquad \left( \because i=\frac{q}{\tau} \right)$

$J=\frac{N \: e }{A \tau} \qquad \left( \because q=Ne \right)$

Now multiply by length of conductor $l$ in above equation. Therefore we get

$J=\frac{N \: e \: l}{A \tau\: l} $

$J=\frac{N \: e \: l}{V \tau\: }\qquad \left(\because V=A.l \right)$

Where $V$ is volume of the conductor.

$J=\frac{n \: e \: l}{ \tau }\qquad \left( \because n=\frac{N}{V} \right)$

Where $n$ is total number of electrons per unit .

$J=n \: e \: v_{d}\qquad \left( \because v_{d}=\frac{l}{\tau}\right)$

Where $v_{d}$ are known as drift velocity of charged particles.

Now substitute the value of current density $J$ from equation $(1)$ to above equation then above equation can be written as

$i=neAv_{d}$

Read More

Energy Stored in a Charged Capacitor

Description→

When a capacitor is charged, the work is done by charged battery (i.e the chemical energy of the battery is used to charge the capacitor). As the capacitor gets charged, the potential difference between its plates increases. Due to this increase in the potential difference between the plates, the battery has to give the same amount of charge to the capacitor. Because of that, the battery has to do more and more work.

The total amount of work done in charging the capacitor is stored in the form of electric potential energy in between the capacitor plates. This energy is retrieved as heat when the capacitor is discharged through a resistance.

Derivation→

Let us consider, a capacitor of capacitance $C$with a potential difference of $V$ between the plates.

In the process of charging, electrons are transferred from the positive to negative, unit each plate acquires an amount of charge $q$. Suppose during the process of charging, we increase the charge from $q'$ to $q'+dq'$ by transferring an amount of negative charge $dq'$ from the positive to the negative plate. the work done

$dw=V' \: dq'$

$dw=\frac{q'}{C} \: dq'$

Therefore, the total work done in charging the capacitor from the uncharged state (i.e. zero charge in the capacitor) to the final charge $q$ will be

$W=\int_{0}^{W} dw$

$W=\int_{0}^{q} \frac{q'}{C} \: dq'$

$W=\frac{1}{2}\left[ \frac{q'^{2}}{C} \right]^{q}_{0} $

$W=\frac{1}{2}\left[ \frac{q^{2}}{C} \right]$

$W=\frac{1}{2}\:C \: V^{2}$

This work done is stored in the form of potential energy $U$ within the capacitor. Thus

$U=\frac{1}{2}\:C \: V^{2} $

$U=\frac{1}{2}\frac{q^{2}}{C}$

Energy Stored in Combination of Capacitor→

If many capacitors are combined in series, or in parallel, the total potential energy stored in either combination is equal to the sum of the potential energies stored in the individual capacitor. This follows from the combination of the capacitor's expressions:

The potential energy is stored in a series combination→

The potential energy stored in a series combination (here $q$ is constant) is

$U=\frac{1}{2}\frac{q^{2}}{C}$

For series combination the capacitance of capacitor i.e. $\frac{1}{C}=\frac{1}{C_{1}}+\frac{1}{C_{2}}+\frac{1}{C_{2}}+..........$. Now substitute the value of $\frac{1}{C}$ in above equation

$U=\frac{1}{2}q^{2} \frac{1}{C_{1}}+\frac{1}{C_{2}}+\frac{1}{C_{2}}+.......... $

$U= \left( \frac{1}{2}q^{2} \frac{1}{C_{1}}+\frac{1}{2}q^{2} \frac{1}{C_{2}}+ \frac{1}{2}q^{2} \frac{1}{C_{2}}+.......... \right)$

$U=U_{1}+U_{2}+U_{3}+.........$

The potential energy is stored in a parallel combination→

The potential energy is stored in a parallel combination (here $V$ is constant) is

$U=\frac{1}{2}CV^{2}$

For parallel combination the capacitance of capacitor i.e. $C=C_{1}+C_{2}+C_{3}+......$. Now substitute the value of $C$ in the above equation

$U=\frac{1}{2} \left( C_{1}+C_{2}+C_{3}+........... \right) V^{2}$

$U= \frac{1}{2} C_{1} V^{2} + \frac{1}{2} C_{2} V^{2} +\frac{1}{2} C_{3}V^{2} +..... $

$U=U_{1}+U_{2}+U_{3}+......$

Read More

Force between the plates of a Charged Parallel Plate Capacitor

Derivation and Description→

Consider, A parallel plate capacitor with a charge $+q$ on one of its plates and $-q$ on the other plate. Let initially the plates of the capacitor are almost, but not quite touching. Due to opposite polarity, there is an attractive force $F$ between the plates. Now If these plates are gradually pulling and apart to a distance $d$, in such a way that $d$ is still small compared to the linear dimension of the plates, then the approximation of a uniform field between the plates is maintained and thus the force remains constant.

The force between the charged parallel plates of the capacitor

Now the work done in separating the plates from near $0$ to $d$,

$W=F.d \qquad(1)$

This work done $(W)$ is stored as electrostatic potential energy $(U)$ between the plates, i.e.

$U=\frac{1}{2}q V $

But $V=E.d$, then the above equation can be written as

$U=\frac{1}{2}q \: E \: d \qquad(2)$

But the equation $(1)$ and equation $(2)$ both are equal then

$F.d=\frac{1}{2}q \: E \: d$

$ F=\frac{1}{2}q \: E $

The factor $\frac{1}{2}$ arises because just outside the conducting plates the field is $E$ and inside the plates, the field is zero. So the average value $\frac{E}{2}$ contributes to the force.

Read More

Capacitance of a Parallel Plate Capacitor Partly Filled with Dielectric Slab between Plates

Derivation→ Let us consider,

The charge on a parallel-plate capacitor = $q$
The area of parallel-plate = $A$
The distance between the parallel-plate = $d$
The dielectric constant of the slab of a material =$K$
The thickness of the material =$t$
The vacuum (or air) between the plates =$(d-t)$ The surface charge density on the plates= $\sigma$

The capacitance of Parallel Plate Capacitor with Dielectric Slab between Plates

The electric field in the air between the plates is

$E_{\circ}=\frac{\sigma}{\epsilon_{\circ}}$

$E_{\circ}=\frac{q}{\epsilon_{\circ}\: A} \qquad(1)$

The electric field in the dielectric material

$E=\frac{q}{\epsilon_{\circ}\: K\: A} \qquad(2)$

The potential difference between the plates

$V=E_{\circ}\left( d-t \right)+E\:t \qquad(3)$

Now substitute the value of $E_{\circ}$ and $E$ in the equation $(3)$, Then we get

$V=\frac{q}{\epsilon_{\circ}\: A} \left( d-t \right)+ \frac{q}{\epsilon_{\circ}\: K\: A} \:t $

$V=\frac{q}{\epsilon_{\circ}\: A} \left[ \left( d-t \right)+ \frac{t}{K} \right] \qquad$

The capacitance of the capacitor is

$C=\frac{q}{V}$

Now substitute the value of electric potential $V$ in the above equation then

$C= \frac{q}{\frac{q}{\epsilon_{\circ}\: A} \left[ \left( d-t \right)+ \frac{t}{K} \right]}$

$C= \frac{\epsilon_{\circ}\: A}{\left[ \left( d-t \right)+ \frac{t}{K} \right]} $

Since $K>1$, the 'effective' distance between the plates becomes less than $d$ and so the capacitance increases.

Special Cases→

1. When the dielectric slab is completely filled between the parallel plates i.e. $t=d$ then the capacitance between the parallel plates
   
   
   $C= \frac{K \: \epsilon_{\circ}\: A}{d}$


2. When there is a vacuum (or air) between the parallel plates i.e. $t=0$, then the capacitance between the parallel plates
   
   
   $C_{\circ}= \frac{ \epsilon_{\circ}\: A}{d}$


3. When there is a slab of metal whose dielectric constant is infinity (K=∞). If the thickness of the slab is t between the parallel plates, then the capacitance between the parallel plates
   
   
   $C= \frac{ \: \epsilon_{\circ}\: A}{d-t}$


4. When the slabs of dielectric constants $K_{1},K_{2},K_{3},K_{4}...........$ and respective thickness $t_{1},t_{2},t_{3},t_{4},........$ is placed in the entire space between the parallel-plates, then the capacitance between the plates
   
   
   $C=\frac{\epsilon_{\circ} A}{d- \left( t_{1}+t_{2}+t_{3}+.... \right)+ \left( \frac{t_{1}}{K_{1}}+\frac{t_{2}}{K_{2}}+\frac{t_{3}}{K_{3}}+....... \right)}$
   
   
   But $d=t_{1}+t_{2}+t_{3}+....$
   
   
   $C=\frac{\epsilon_{\circ} A}{ \left( \frac{t_{1}}{K_{1}}+\frac{t_{2}}{K_{2}}+\frac{t_{3}}{K_{3}}+..... \right)}$

Read More

Parallel Plate Air Capacitor and Its Capacitance

Parallel Plate Air Capacitor→

A parallel-plate capacitor consists of two long, plane, metallic plates mounted on two insulating stands and placed at a small distance apart in a vacuum (or air). The plates are exactly parallel to each other.

Parallel Plate Air Capacitor

Derivation of the capacitance of parallel plate capacitor in the air→

Let us consider, Two plates $X$ and $Y$ are separated at a small distance $d$ in the vacuum (or air). If the area of plates is $A$ and the plates $X$ and $Y$ have charge $+q$ and $-q$ respectively. If the surface charge density on each plate is $\sigma$ then electric field intensity at a point between two parallel plates is

$E=\frac{\sigma}{\epsilon_{\circ}}$

$E=\frac{q}{\epsilon_{\circ}A}\qquad(1) \qquad (\because \sigma=\frac{q}{A}) $

The potential difference between the parallel plates capacitor in air (or vacuum) is

$V=E.d$

Now substitute the value of $E$ from equation$(1)$ in the above equation

$V=\frac{q}{\epsilon_{\circ}A}.d \qquad(2)$

The capacitance of the parallel plate capacitor in air (or vacuum) is

$C_{\circ}=\frac{q}{V} \qquad(3)$

From equation $(2)$ and equation $(3)$

$C_{\circ}=\frac{q}{\frac{q}{\epsilon_{\circ}A}.d}$

$C_{\circ}=\frac{\epsilon_{\circ}A}{d}$

If the space between the plates is filled with some dielectric medium of dielectric constant $K$, then the electric field between the plates is

$E=\frac{\sigma}{K \: \epsilon_{\circ}}$

The Potential difference between the parallel plates when dielectric medium $(K)$ is present between them

$V=\frac{q}{K \: \epsilon_{\circ} \: A}.d $

Now, The capacitance of the parallel-plate capacitor when the dielectric medium $(K)$ is present between them

$C=\frac{K\: \epsilon_{\circ} \: A}{d}$

Read More

Potential Energy of a Charged Conductor

Definition:

The work done in charging the conductor is stored as potential energy in the electric field in the vicinity of the conductor is called the potential energy of a charged conductor.

Derivation →

Let us consider, a conductor of capacitance $C$. If charge $+Q$ is given into small amount of $dq$ to the surface of the conductor. Then the work done will be

$dw=V\:dq \qquad(1) $

$dw=\frac{q}{C} \:dq \qquad \left (\because V=\frac{q}{C} \right)$

Therefore, as the amount of charge on the conductor will increase from $0$ to $Q$ that causes also increase in work done. So integrate the above equation for total work done

$ \int_{0}^{W} dw=\frac{1}{C} \int_{0}^{Q} q dq$

$W=\frac{1}{C} \int_{0}^{Q} q dq$

$W=\frac{1}{C} \left[ \frac{q^{2}}{2} \right]^{Q}_{0}$

$W=\frac{1}{2} \frac{Q^{2}}{C}$

This work is stored in the form of electric potential energy $U$. Then

$U=\frac{1}{2} \frac{Q^{2}}{C}$

$U=\frac{1}{2} C V^{2}$

$U=\frac{1}{2} Q V$

Read More

Capacitance of an Isolated Spherical Conductor

Derivation →

Capacitance of an Isolated Spherical Conductor

Let us consider an isolated spherical conductor of radius $a$ is placed in a vacuum or air. Let a charge $+q$ be given to the sphere and this charge is distributed uniformly on the surface of conducting sphere. Then the electric potential at the surface of the conducting sphere is

$V=\frac{1}{4\pi \epsilon_{\circ}} \frac{q}{a} \qquad(1)$

So the capacitance of the sphere

$C=\frac{q}{V} \qquad(2)$

Now substitute the value of $V$ in equation $(2)$ Then we get

$C=\frac{q}{\frac{q}{4\pi \epsilon_{\circ}a}}$

$C=4\pi \epsilon_{\circ}a$

Thus, The capacitance of a spherical conductor is directly proportional to its radius. i.e If the radius of conducting sphere is large then the sphere will hold a large amount of the given charge without running up too high a voltage.

Unit: The unit of capacitance is "Farad" i.e. $F$

Read More

Magnetic Field at the axis of a current-carrying Circular Loop

Derivation→

Let us consider, A circular loop which have radius $a$, carrying a current $i$. Let take a point $P$ on the axis of the loop at a distance $x$ from the center $O$ of the loop.

Now to find the magnetic field at point $P$, take a small current element of length $dl$ at the top of the loop. Let $r$ is the distance between the current element and point $P$. Now apply the Biot-Savart's Law to find the magnitude of the magnetic field due to small current-element $dl$ at point $P$ i.e.

$dB=\frac{\mu_{\circ}}{4 \pi} \frac{i \: dl\: sin\theta}{r^{2 }}$

Where $\theta$ is the angle between the length of the element $dl$ and the line joining the element to the point $P$. Here this angle is $90^{\circ}$

$dB=\frac{\mu_{\circ}}{4\pi} \frac{i \: dl}{r^{2}}\qquad(1)$

The direction of the magnetic field $d\overrightarrow{B}$ is in the plane of the paper and at right angles to the line $r$ as shown in the figure below (By applying the right-hand screw rule). It can be resolved into two components, one is vertical component of magnitude $dB\: sin\phi$ along the axis of the loop and the other is horizontal component of magnitude $dB\: cos\phi$ at right angles to the axis.

Magnetic Field at the Axis of a Current-Carrying Circular Loop

Let us consider another current element of the same length at the bottom of the loop, directly opposite to the first element, which is at right angles to the plane of the page but directed inwards. the field due to $d\overrightarrow{B}$ but directed as shown in the figure above. It is clear that components of both the fields at right angles to the axis are equal and opposite. Hence they cancel each other. The component along the axis are in the same direction and so they are added up.

If we imagine the whole of the loop to be divided into such current elements, the resultant field $\overrightarrow{B}$ at $P$ is directed along the axis and its magnitude is given by

$B=\int dB \: \sin\phi$

Now substitute the value of $dB$ from equation $(1)$

$B=\int \frac{\mu_{\circ}}{4\pi} \frac{i \: dl}{r^{2}} \: \sin\phi$

From figure, $sin\phi=\frac{a}{r}$, we get

$dB=\int \frac{\mu_{\circ}}{4 \pi} \frac{i}{r^{2}} \frac{a}{r}dl$

$dB=\int \frac{\mu_{\circ}}{4 \pi} \frac{ia}{r^{3}} dl$

$dB= \frac{\mu_{\circ}}{4 \pi} \frac{ia}{r^{3}} \int dl$

$dB= \frac{\mu_{\circ}}{4 \pi} \frac{ia}{r^{3}} 2 \pi \: a \qquad \left( \int dl = 2 \pi \: a \right)$

$dB= \frac{\mu_{\circ}ia^{2}}{2r^{3}}$

Agian from figure $r^{2}=\left( a^{2} + x^{2}\right)^{3}$ or $r= \left( a^{2} + x^{2}\right)^{3/2}$. Now substitute the value of $r$ in above equation.

$B=\frac{\mu_{\circ}\: i\: a^{2}}{2(a^{2}+x^{2})^{3/2}}$

If it is a coil of $N$ turns, then each turn will contribute equally to $B$. Thus

$B=\frac{\mu_{\circ}\: N \: i \: a^{2}}{2(a^{2}+x^{2})^{3/2}}$

The direction of the magnetic field $\overrightarrow{B}$ is along the axis of the loop and that of the coil.

Magnetic field at the Centre of the Loop→

At the centre of the coil, we have $x=0$

$B=\frac{\mu_{\circ}\: N \: i}{2a}$

Again the direction of the magnetic field $\overrightarrow{B}$ is perpendicular to the plane of the coil i.e. Along the axis of the coil.

Read More

Magnetic Field due to a Straight Current-Carrying Conductor of Finite Length

Derivation→

Let us consider, a current-carrying conductor $XY$ having length $l$ in which current $i$ is flowing from $X$ to $Y$. Now, To find the magnetic field due to the conductor, take a point $P$ at a distance $d$ from point $O$ of the conductor.

Now consider a small length element $dl$ at the conductor which is making an angle $\theta$ from point $P$. The length element $dl$ is also making the angle $d\theta$ from point $O$. If $\theta_{1}$ and $\theta_{2}$ is the angle from point $O$ to point $X$ and $Y$ respectively. Than magnetic field at point $P$ due to small length element $dl$ which is at a distance $r$

$dB=\frac{\mu_{0}}{4\pi} \frac{i\: dl \: sin (90+\theta)}{r^{2}}$

$dB=\frac{\mu_{0}}{4\pi} \frac{i \: dl \: cos\theta}{r^{2}} \qquad(1)$

Magnetic Field due to a Straight Current-Carrying Conductor of Finite Length

But from the figure, In $\Delta NOP$

$cos\theta=\frac{d}{r}$

$r=\frac{d}{cos\theta} \qquad(2)$

$tan\theta=\frac{l}{d}$

$l=d\:tan\theta$

Now differentiate the above equation with respect to $\theta$, then

$dl=d \: sec^{2}\theta \: d\theta \qquad(3)$

Now substitute the value of $dl$ and $r$ from equation$(2)$ and equation $(3)$ in equation$(1)$, then we get

$dB=\frac{\mu_{o}i}{4\pi}\frac{d \:sec^{2}\theta \: d\theta \: cos\theta}{\frac{d^{2}}{cos^{2}\theta}}$

$dB=\frac{\mu_{o}i}{4\pi}\frac{sec^{2}\theta \: d\theta \: cos^{3}\theta}{d}$

$dB=\frac{\mu_{o}i}{4\pi}\frac{cos\theta \: d\theta}{d} \qquad(4)$

The magnitude of the magnetic field $\overrightarrow{B}$ at point $P$ due to the whole conductor $XY$ is

$B=\int_{-\theta_{1}}^{\theta_{2}}dB$

Now substitute the value of $dB$ in above equation so

$B=\int_{-\theta_{1}}^{\theta_{2}} \frac{\mu_{o}i}{4\pi}\frac{cos\theta \: d\theta}{d} $

$B=\int_{-\theta_{1}}^{\theta_{2}} \frac{\mu_{o}i}{4\pi d}cos\theta d\theta $

$B= \frac{\mu_{o}i}{4\pi d} \left[ sin\theta\right] _{-\theta_{1}} ^{\theta_{2}} $

$B= \frac{\mu_{o}i}{4\pi d} \left[ sin(\theta _{2}) - sin(-\theta_{1})\right] $

$B=\frac{\mu_{o}i}{4\pi d}\left( sin\theta _{1} + sin\theta_{2}\right) $

Special Case→

1. For a conductor of infinite length, i.e $\theta_{1}=\theta_{2}=90^{\circ}$ then magnetic field
   
   
   $B=\frac{\mu_{0}}{4\pi} \frac{2i}{d} $
   
   
   $B=\frac{\mu_{0}}{2\pi} \frac{i}{d} $


2. If the point $P$ is at a distance $d$ near one end of the conductor,i.e. $\theta_{1}=90^{\circ}$ and $\theta_{2}=0$ then magnetic field
   
   
   $B=\frac{\mu_{0}}{4\pi} \frac{i}{d} $
   
   
   From the above equations, we can conclude that the magnetic field $B$ at point $P$ is proportional to the current $i$ and inversely proportional to the distance $d$ of point $P$ from the conductor.

The variation of the magnitude of magnetic field $B$ with distance $d$ is shown in the figure below→

Variation in Magnetic field with distance

The line of force of magnetic field $\overrightarrow{B}$ near a linear current-carrying conductor are concentric circles of varying radii with their centers at the conductor in a plane perpendicular to the conductor. The direction of the magnetic field $\overrightarrow{B}$ at a point $P$, distant $d$, will be along the tangent drawn on a circle of radius $d$ around the conductor.

Line of force of Magnetic field near Current-Carrying Conductor

Read More

Motion of Charged Particles in Uniform Magnetic Field

Description:

The motion of any particle is depends upon the force applying on it. In the magnetic field, the motion of charge is perpendicular to the magnetic force applied on the charge particle because of that the path of the charge particle becomes circular. If the direction of force and the direction of motion are not perpendicular to each other that is they are at any angle then the path of the charge particle becomes becomes helical.

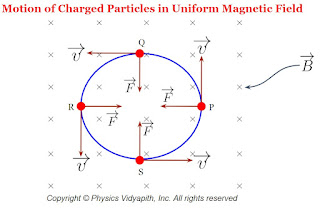
A.) When $\overrightarrow{v}$ is perpendicular to $\overrightarrow{B}$→

Let us consider a charge $q$ enters in the magnetic field $\overrightarrow{B}$ from a point $O$ with velocity $\overrightarrow{v}$ directed perpendicular to the magnetic field $\overrightarrow{B}$.The general Expression for the force acting on the particle is

$\overrightarrow{F}=q(\overrightarrow{v} \times \overrightarrow{B})$

Here the magnetic field $\overrightarrow{B}$ is perpendicular to the plane of the page directed downwards which is shown in the figure below.

Since the velocity of charge particle $\overrightarrow{v}$ is perpendicular to the magnetic field $\overrightarrow{B}$ then the magnitude of the force $F$ will be

$F=qvB \qquad(1)$

Since the force is perpendicular to the velocity and the direction of velocity continuously changes but the magnitude of velocity is not changing. Therefore the particle will move in a circular path with constant speed in a uniform magnetic field and the magnetic force will act as a centripetal force. i.e.

$F=\frac{m v^{2}}{r} \qquad(2)$

Where
$r$ → Radius of the circular path
$m$ → mass of the particle

From equation $(1)$ and equation $(2)$

$\frac{m v^{2}}{r}=qvB$

$r=\frac{mv}{qB}$

The direction of the path of the particle depends upon the nature of the charge and the direction of the magnetic field.

The particle travels a distance $2\pi r$ in one revolution. So the time period of one revolution is

$T=\frac{2\pi r}{v} \qquad(3)$

Now substitute the value of $r=\frac{mv}{qB}$ in equation $(3)$ then we get

$T=\frac{2\pi m}{qB}$

The frequency of the particle $n=\frac{1}{T}$ can be written as

$n=\frac{qB}{2\pi m}$

From the above equations, We can conclude that the time period or frequency of the particle is independent of the speed $v$ of the particle. If the speed of the charge particle increases, its radius also increases so that the time taken to complete one cycle or revolution remains the same. If two identical charged particles enter the field with different speeds $v_{1}$ and $v_{2}$, then they move along the circle of smaller and larger radii respectively which is shown in the figure below.

The frequency and Time period of the particle are independent of the speed

B.) When $\overrightarrow{v}$ is not perpendicular to $\overrightarrow{B}$→

Now, Again Let that the particle is entering with velocity $\overrightarrow{v}$ in the magnetic field $\overrightarrow{B}$, instead of being perpendicular to $\overrightarrow{B}$, makes angle $\theta (0 < \theta < 90^{\circ})$ with it. Now the velocity $\overrightarrow{v}$ may resolved into two components;

First is:

$v_{\parallel}=v \: cos\theta$ i.e. Parallel to magnetic field $\overrightarrow{B}$. This component gives the linear path to the particle.

Second is:

$v_{\perp}=v \: sin\theta$ i.e. Perpendicular to magnetic field $\overrightarrow{B}$. This component gives the circular path to the particle.

The resultant of these two-component gives the helical path to the particle whose axis is parallel to the magnetic field.

The radius of the circular path of the helix is

$r=\frac{mv_{\perp}}{qB}$

$r=\frac{mv \: sin\theta}{qB}$

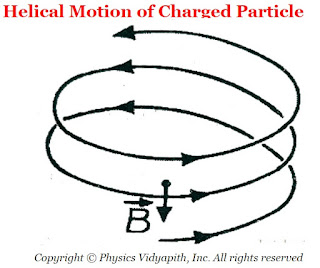Helical Motion of Charged Particle

The time period of the particle

$T=\frac{2\pi m}{qB}$

The linear distance traveled by the particle in the direction of the magnetic field in one complete circle is called the 'pitch $(p)$' of the path.

$p=v_{\parallel} \times T$

$T= v \: cos\theta \times \frac{2\pi m}{qB}$

Kinetic Energy of Charged Particle Moving in Uniform Magnetic Field→

The kinetic energy is

$K=\frac{1}{2}mv^{2}$

$K=\frac{(mv)^{2}}{2m}$

Now substitute the value of $mv$ from $r=\frac{mv}{qB}$. Then we get

$K=\frac{1}{2}m \left( \frac{rqB}{m} \right)^{2}$

$K=\frac{r^{2}q^{2}B^{2}}{2m}$

Read More

Normalized and Orthogonal wave function

Description:

We know that $\psi^{*}\psi$ or $\left|\psi \right|^{2} d\tau $ represent the probability of finding the particle in volume element $d\tau$.

The total probability of finding the particle in the entire space is 1 so

$ \int \left|\psi(r,t) \right|^{2} d\tau=1 $

Where integral extends overall space.

$\int \psi^{*}(r,t) \psi(r,t) d\tau=1$

A wave function satisfies the above equation so it is called normalized to unity. For any wave function that is a solution of the time-dependent Schrodinger equation

$\int \psi^{*} \psi d\tau=N$

$\frac{1}{N} \int \psi^{*} \psi d\tau=1$

$\int \frac{\psi{*}}{\sqrt{N}} \frac{\psi}{\sqrt{N}} d\tau = 1$

Where
$\sqrt{N}$ → Normalized Factor
$\frac{\psi}{N} $ → Normalised wave function

If independent coordinate $x$,$y$,$z$, and $\psi$ satisfy the Schrodinger wave equation. Then it is evident that $\frac{\psi}{\sqrt {N}}$ also satisfies the Schrodinger wave equation.

If $\psi_{i}$ and $\psi_{j}$ are two different wave functions both the satisfactory solution of the wave equation for a given system. Then these functions will be normalized if

$\psi_{i}^{*} \psi_{i} d\tau=1 \quad and \quad \psi_{j}^{*} \psi_{j} d\tau=1$

If the two wave function $\psi_{i}$ and $\psi_{j}$ are the satisfactory solution of the wave equation for a given system. Then these functions will be mutually orthogonal if

$\psi_{i}^{*} \psi_{j} d\tau=0 \qquad Where \: $i \neq j$

$\psi_{j}^{*} \psi_{i} d\tau=0 \qquad Where \: $i \neq j$

These integral vanishes over the entire space.

Read More