Power in Alternating Current Circuit

Definition of Power in Alternating Current Circuit:

The rate of power consumption in an alternating current circuit is known as power in the circuit.

Mathematical Analysis:

Let us consider an alternating current circuit in which the voltage and current at any instant are given by

$V=V_{\circ} sin \omega t \qquad(1)$

$i=i_{\circ} sin \left( \omega t - \phi \right) \qquad(2)$

Where $\phi$ $\rightarrow$ Phase difference between voltage and current

So instantaneous Power

$P=Vi$

$P=\left\{V_{\circ} sin \omega t \right\} \left\{ i_{\circ} sin \left( \omega t - \phi \right) \right\}$

$P=V_{\circ} i_{\circ} \: sin \omega t \: sin \left( \omega t - \phi \right)$

$P=\frac{V_{\circ} i_{\circ}}{2} \left[2\: sin \: \omega t \: sin \left( \omega t - \phi \right)\right]$

$P=\frac{V_{\circ} i_{\circ}}{2} \left[ cos \left( \omega t -\omega t + \phi \right) \\ \qquad - cos \left( \omega t + \omega t - \phi \right) \right]$

$P=\frac{V_{\circ} i_{\circ}}{2} \left[ cos \left(\phi \right) - cos \left( 2\omega t - \phi \right) \right]$

The average power for one cycle is:

$P_{avg}$ = The average value of $\left\{ \frac{V_{\circ} i_{\circ}}{2} \left[ cos \left(\phi \right) - cos \left( 2\omega t - \phi \right) \right] \right\}$

The term $cos \left( 2\omega t - \phi \right)$ is time-dependent and the average value of term $cos \left( 2\omega t - \phi \right)$ for complete one cycle is zero. So the average power dissipation in one cycle is

$P_{avg}= \frac{V_{\circ} i_{\circ}}{2} cos \phi $

$P_{avg}= \frac{V_{\circ}}{\sqrt{2}} \frac{i_{\circ}}{\sqrt{2}} cos \phi $

$P_{avg}= V_{rms} \: i_{rms} cos \phi $

Case -1 The circuit containing Pure Resistor only:

If the circuit contains a pure resistance only then voltage $V$ and current $i$ are always in the same phase. i.e. $\phi=0^{\circ}$ and $cos \phi = +1$, then average power

$P_{avg}= V_{rms} \: i_{rms} $

Case -2 The circuit containing Pure Inductor only:

If the circuit containing pure inductor only then voltage $V$ leads over current $i$ by $90^{\circ}$ i.e. $\phi=90^{\circ}$ and $cos 90^{\circ} = 0$, then average power

$P_{avg}= 0 $

Case -3 The circuit containing Pure Capacitor only:

If the circuit containing pure capacitor only then voltage $V$ lags behind current $i$ by $90^{\circ}$ i.e. $\phi=90^{\circ}$ and $cos 90^{\circ} = 0$, then average power

$P_{avg}= 0 $

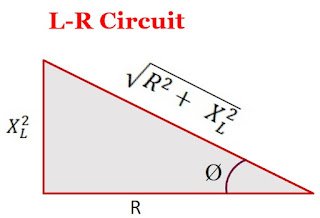
Case -4 The circuit containing inductor and resistor only (L-R Circuit):

We know that the phase in the L-R circuit is

$tan \phi = \frac{X_{L}}{R}$

From the above equation, Draw the triangle as shown in the figure below:

From the above figure, the value of $cos\phi$ is

$cos \phi = \frac{R}{\sqrt{R^{2} + X^{2}_{L}}}$

So average power

$P_{avg}= V_{rms} \: i_{rms} \frac{R}{\sqrt{R^{2} + X^{2}_{L}}} $

$\left(P_{avg}\right)_{L-R} \lt \left(P_{avg}\right)_{R}$

Thus, the average power consumption in an L-R circuit is less than the power consumed in a purely resistive circuit

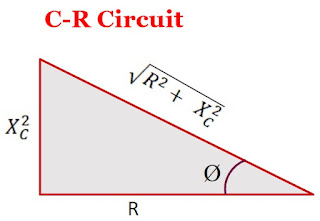
Case -5 The circuit containing capacitor and resistor only (C-R Circuit):

We know that the phase in the C-R circuit is

$tan \phi = \frac{X_{C}}{R}$

From the above equation, Draw the triangle as shown in the figure below:

From the above figure, the value of $cos\phi$ is

$cos \phi = \frac{R}{\sqrt{R^{2} + X^{2}_{C}}}$

So average power

$P_{avg}= V_{rms} \: i_{rms} \frac{R}{\sqrt{R^{2} + X^{2}_{C}}} $

$\left(P_{avg}\right)_{C-R} \lt \left(P_{avg}\right)_{R}$

Thus, the average power consumption in a C-R circuit is less than the power consumed in a purely resistive circuit

Case -6 The circuit containing capacitor and inductor only (L-C Circuit):

We know that the phase in the L-R circuit is

$\phi = 90^{\circ}$ and $cos 90^{\circ} = 0$

So average power

$P_{avg}= 0 $

Case -7 The circuit containing inductor, capacitor, and resistor only (L-C-R Circuit):

We know that the phase in the L-C-R circuit is

$tan \phi = \frac{X_{L} - X_{C}}{R}$

From the above equation, Draw the triangle as shown in the figure below:

From the above figure, the value of $cos\phi$ is

$cos \phi = \frac{R^{2}}{\sqrt{R^{2} + \left( X^{2}_{L} - X^{2}_{C} \right) }}$

So average power

$P_{avg}= V_{rms} \: i_{rms} \frac{R^{2}}{\sqrt{R^{2} + \left( X^{2}_{L} - X^{2}_{C} \right) }} $

$\left(P_{avg}\right)_{L-C-R} \lt \left(P_{avg}\right)_{R}$

Thus, the average power consumption in an L-C-R circuit is less than the power consumed in a purely resistive circuit

Wattless Current:

If the circuit contains only resistor or only inductor or only inductor and capacitor then power consumption in the circuit will be zero (i.e. there will be no energy dissipation in the circuit) due to the phase difference $90^{\circ}$ between voltage(or impedance) and current. So the current in the circuit is called the "Wattless current".

Read More

Self Induction Phenomenon and its Coefficient

Self Induction:

When a changing current flows in a coil then due to the change in magnetic flux in the coil produces an electro-motive force $\left(emf \right)$ in that coil. This phenomenon is called the principle of Self Induction.

The direction of electro-motive force can be found by applying "Lenz's Law".

Mathematical Analysis of Coefficient of Self Induction:

Let us consider that a coil having the number of turns is $N$. If the change in current is $i$, then linkage flux in a coil will be

$N \phi \propto i$

$N\phi = L i \qquad(1)$

Where $L$ $\rightarrow$ Coefficient of Self Induction.

According to Faraday's law of electromagnetic induction. The electro-motive force $\left(emf \right)$ in a coil is

$e=-N\left( \frac{d \phi}{dt} \right)$

$e=-\frac{d \left(N \phi \right)}{dt} \qquad(2)$

From equation $(1)$ and equation $(2)$

$e=-\frac{d \left(L i\right)}{dt} $

$e=-L \left(\frac{d i}{dt} \right) $

$L = \frac{e}{\left(\frac{d i}{dt} \right)}$

If $\left(\frac{d i}{dt} \right) = 1$

Then

$L = e$

The above equation shows that If the rate of flow of current in a coil is unit then the coefficient of self-induction in that coil will be equal to the induced electro-motive force $\left( emf \right)$.

Read More

Mutual Induction Phenomenon and its Coefficient

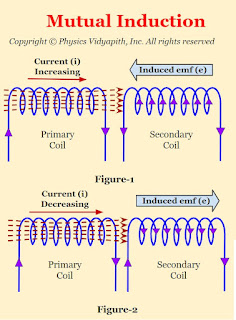
Mutual Induction:

When two coils are placed near each other then the change in current in one coil ( Primary Coil) produces electro-motive force $\left( emf \right)$ in the adjacent coil ( i.e. secondary coil). This phenomenon is called the principle of Mutual Induction.

The direction of electro-motive force $\left( emf \right)$ depends or can be found by "Lenz's Law"

Mathematical Analysis of Coefficient of Mutual Induction:

Let us consider that two coils having the number of turns are $N_{1}$ and $N_{2}$. If these coils are placed near to each other and the change in current of the primary coil is $i_{1}$, then linkage flux in the secondary coil will be

$N_{2}\phi_{2} \propto i_{1}$

$N_{2}\phi_{2} = M i_{1} \qquad(1)$

Where $M$ $\rightarrow$ Coefficient of Mutual Induction.

According to Faraday's law of electromagnetic induction. The electro-motive force $\left( emf \right)$ in the secondary coil is

$e_{2}=-N_{2}\left( \frac{d \phi_{2}}{dt} \right)$

$e_{2}=-\frac{d \left(N_{2} \phi_{2} \right)}{dt} \qquad(2)$

From equation $(1)$ and equation $(2)$

$e_{2}=-\frac{d \left(M i_{1} \right)}{dt} $

$e_{2}=-M \left(\frac{d i_{1}}{dt} \right) $

$M = \frac{e_{2}}{\left(\frac{d i_{1}}{dt} \right)}$

If $\left(\frac{d i_{1}}{dt} \right) = 1$

Then

$M = e_{2}$

The above equation shows that If the rate of flow of current in the primary coil is unit then the coefficient of mutual induction will be equal to the induced electro-motive force $\left( emf \right)$ in the secondary coil.

Read More

Faraday's laws of electromagnetic induction

Faraday's Laws of Electromagnetic Induction:

The Faraday's experiment shows the two laws which are known as Farday's laws of electromagnetic induction

First Law (Neumann's Law): The rate of change of magnetic flux through a circuit is equal to the emf produced in the circuit. This is also known as "Neumann Law"

$e=-\frac{\Delta \phi}{ \Delta t}$

Here negative sign shows the direction of emf.

If $\Delta t \rightarrow 0$

$e=-\frac{d \phi}{ d t}$

This equation represents an independent experimental law that cannot be derived from other experimental laws.

If the circuit is a tightly wound coil of $N$ turns, then the induced emf

$e=-N\frac{d \phi}{ d t}$

$e=-\frac{d \left(N \phi\right)}{ dt}$

Here $N \phi$ is called the 'Linkage magnetic flux'.

Note: The change in flux induces emf, not the current.

Second Law (Lenz's Law): The direction of induced EMF produced in a closed circuit is such that it opposes the original cause that produces it. It is also called "Lenz's law". The direction of induced EMF is described by Fleming's right-hand rule.

Read More

Paramagnetic Substances and Its properties

Paramagnetic Substances :

Those substances, which are placed in the external magnetic field and they are weakly magnetized in the direction of the external magnetic field, are called paramagnetic substances. The susceptibility $\chi_{m} $ of paramagnetic substances is small and positive. Further, When a paramagnetic substance is placed in the magnetic field, then the flux density of the paramagnetic substance is slightly more than the free space. Thus, the relative permeability of paramagnetic substance $\mu_{r}$, is slightly more than 1.

Properties of Paramagnetic substances:

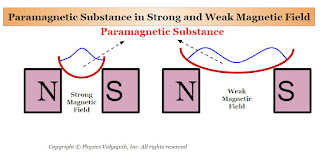
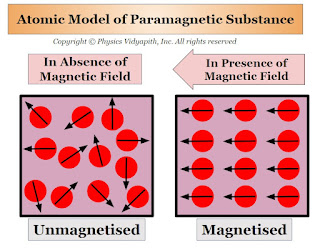
1. When a rod of a paramagnetic material is suspended freely between external magnetic poles (i.e. Between North and South Poles) then its axis becomes along the direction of the external magnetic field $B$ (Figure). The poles produced on the two sides of the rod are opposite to the poles of the external magnetic field.

2. In a non-uniform magnetic field, a paramagnetic substance tends to move from the weaker magnetic field to the stronger magnetic field. If a paramagnetic liquid is taken in a watch glass placed on two magnetic poles very near to each other, then the liquid rises in the middle as shown in the figure below(Figure) where the field is strongest. Now, if the distance between the poles is increased, the liquid is depressed in the middle, because now the field is strongest near the poles.

3. If the solution of a paramagnetic substance is poured into a U-tube and apply the strong magnetic field into one arm of this U-tube then the level of the solution in that arm rises. As shown in the figure below:

4. When paramagnetic gas molecules are passed between the poles of a magnet then paramagnetic gas molecules are attracted toward the magnetic field.

5. The susceptibility of a paramagnetic substance is inversely dependent on temperature.

$\chi \propto \frac{1}{T_{C}-T}$

Explanation of Paramagnetism on the Basis of Atomic Model:

The property of Paramagnetism is generally found in those substances whose atoms (or ions or molecules) have an 'odd' number of electrons. In these odd numbers of electrons one electron is not able to form a pair because the net magnetic dipole moment of the atoms (or ions or molecules) are not zero. but in the absence of an external magnetic field, these magnetic dipole moments are randomly arranged inside the substance because the net magnetic dipole moment of the material is zero.

When a paramagnetic substance is placed in an external magnetic field $B$ then the magnetic dipole moment of the atoms (or ions or molecules) are weakly aligned in the direction of the external magnetic field. Thus, a small magnetic dipole moment is induced in the substance which is directly proportional to the magnetic field $B$. Hence, the paramagnetic substance is magnetized in the direction of the external magnetic field $B$, and the field lines become less dense inside the paramagnetic substance compared to those outside.

Read More

Work energy theorem Statement and Derivation

Work-energy theorem statement:

The work between the two positions is always equal to the change in kinetic energy between these positions. This is known as the work energy Theorem.

$W=K_{f}-K_{i}$

$W=\Delta K$

Derivation of the Work-energy theorem:

According to the equation of motion:

$v^{2}_{B}=v^{2}_{A}+2as $

$2as=v^{2}_{B}-v^{2}_{A}$

$2mas=m(v^{2}_{B}-v^{2}_{A})$

$mas=\frac{m}{2} (v^{2}_{B}-v^{2}_{A})$

$Fs=\frac{1}{2}mv^{2}_{B}-\frac{1}{2}mv^{2}_{A} \qquad (\because F=ma)$

$W=\frac{1}{2}mv^{2}_{B}-\frac{1}{2}mv^{2}_{A} \qquad (\because W=Fs)$

$W=K_{f}-K_{i}$

Where
$K_{f}$= Final Kinetic Energy at position $B$
$K_{i}$= Initial Kinetic Energy at position $A$

$W=\Delta K$

Alternative Method (Integration Method):

We know that the work done by force on a particle from position $A$ to position $B$ is-

$W=\int F ds$

$W=\int (ma)ds \qquad (\because F=ma)$

$W=m \int \frac{dv}{dt}ds$

$W=m \int dv \frac{ds}{dt}$

$W=m \int v dv \qquad (\because v=\frac{ds}{dt})$

If position $A$ is the initial point where velocity is $v_{A}$ and position $B$ is the final point where velocity is $v_{B}$ then work is done by force under the limit-

$W=m \int_{v_{A}}^{v_{B}} v dv$

$W=m[\frac{v^{2}}{2}]_{v_{A}}^{v_{B}} $

$W=\frac{1}{2}mv^{2}_{B}-\frac{1}{2}mv^{2}_{A}$

$W=K_{f}-K_{i}$

$W=\Delta {K}$

Read More

Davisson and Germer's Experiment and Verification of the de-Broglie Relation

Davisson and Germer's Experiment on Electron Diffraction:

Davisson and Germer's experiment verifies the wave nature of electrons with the help of diffraction of the electron beam as wave nature exhibits the diffraction phenomenon.

Principle: The principle of Davisson and Germer's experiment is based on the diffraction phenomenon of the electron beam by crystal and it verifies the de-Broglie relation.

Theoretical Formula: If a narrow beam of electrons is accelerated by a potential difference $V$ volts, the kinetic energy $K$ acquired by each electron in the beam is given by

$K=eV \qquad(1)$

Where $e$ is the charge of an electron

The de-Broglie wavelength is given by

$\lambda = \frac{h}{\sqrt {2m_{\circ} K \left( 1+ \frac{E_{K}}{2m_{\circ}c^{2}} \right)}}$

If $E_{K} \lt \lt 2m_{\circ}c^{2}$, then the term $\frac{E_{K}}{2m_{\circ}c^{2}}$ will be negligible. So above equation can be written as

$\lambda = \frac{h}{\sqrt {2m_{\circ} K}} \qquad(2)$

Now subtitute the value of $K$ from equation $(1)$ to equation $(2)$ then equation $(2)$ can be written as

$\lambda = \frac{h}{\sqrt {2m_{\circ} eV}}$

Now substitute the numerical value of $h$, $m_{\circ}$ and $e$, we get

$\lambda = \frac{6.63 \times 10^{-34}}{2 \times 9.11 \times 10^{-31} \times 1.6 \times 10^{-19} \times V}$

$\lambda = \frac{6.63 \times 10^{-9}}{29.15\times V}$

$\lambda = \frac{12.28}{\sqrt{V}}A^{\circ} \qquad(3)$

This is the theoretical value of $\lambda$ for the known potential difference in volts.

The calculation shows that the wavelength of the waves associated with the beam of electrons is of the same order as that of X-rays. Therefore, if such a beam of electrons is reflected from a crystal, the reflected beam will show the same diffraction and interference phenomena as for X-rays of the same wavelength. This consideration was the basis of Davisson and Germer's experiments. In one set of experiments, the [111] face of the nickel crystal was arranged perpendicular to the incident beam of electrons.

Apparatus:

There are the following apparatus is used in the Davisson and Germer experiment.

1. Electron gun
2. Target
3. Electron detector or Faraday's cylinder
4. Galvanometer

1. Electron gun: The electron gun is a device that is used to produce a highly accelerated and collimated electron beam by applying high potential.

2. Target: It is a single large metal crystal i.e. nickel used as a target. In crystal, the atoms are arranged in regular lattice i.e. [111] so that the surface lattice of the crystal acts as a diffraction grating and the electrons get diffracted by the crystal in different directions. The electron beam is incident normal to the nickel crystal. The crystal can be rotated about an axis perpendicular to the incident beam so that various azimuthal angles could be used.

3. Electron detector or Faraday's cylinder: It is used to detect or measure the intensity of diffracted beams of electrons. It can be moved along a circular scale $S$. This electron detector is connected to a Galvanometer.

4. Galvanometer: The galvanometer is a device that is used to measure the very small amount of current following in the circuit.

The whole apparatus is completely enclosed and highly evacuated.

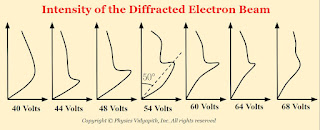
Working: When a low potential is applied to the electron gun so that a beam of slow electrons emerges from the gun and falls normally on the surface of the crystal. To collect the diffracted electrons, the Faraday cylinder is moved to various positions on the scale $S$ and the corresponding galvanometer measures the current at each position through an electron detector. The observation is repeated for electrons accelerated through different potentials. The current which is a measure of the intensity of the diffracted electron beam, is plotted against the diffracting angle $\phi$ for each accelerating potential as shown in the figure below

It is observed in the curves that at the voltage of $40$ volts, a smooth curve is obtained and a bump begins to appear in the curve for $44$ volts. As the potential difference is further increased, the bump starts shifting upward and becomes most prominent in the curve for $54$ volts at $\phi = 50 ^{\circ}$. Beyond $54$ volts the bump gradually diminishes and becomes insignificant at $68$ volts. The pronounced current peak at $54$ volts and at $50^{\circ}$ provided an evidence that electrons were diffracted by the target and verifies the existence of electron wave nature.

Calculation of Wavelength:

Theoretical Calculation:

The wavelength of electron at $54$ volts can be find by de- Broglie formula as shown in the equation $(3)$

$\lambda= \frac{12.28}{\sqrt{54}}\: A^{\circ}$

$\lambda= 1.671 \: A^{\circ} $

Experimental Calculation:

Experimental calculation is done by Bragg's diffraction equation

$n \lambda = 2d \: sin\theta$

Where
$d \rightarrow$ interplanar Spacing
$n \rightarrow$ Order of plane

For nickel crystal :
$n=1$
$d=0.91 A^{\circ}$

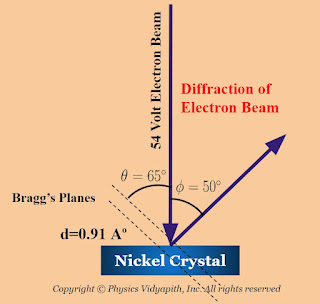
In the experiment, the diffracted electron beam appearing at $\phi=50 ^{\circ}$ aries from wave-like diffraction from the family of Bragg's planes.
The corresponding angle of incidence relative to the family of Bragg's planes is
$\theta = \frac{180 - \phi}{2}$
$\theta = \frac{180 - 50}{2}$
$\theta= 65^{\circ}$

Now apply these values to Bragg's equation as written above

$\lambda = 2 \times 0.91 \times sin 65^{\circ} $

$\lambda = 2 \times 0.91 \times 0.906 $

$\lambda = 1.65 A^{\circ} $

The theoretical and experimental value at $54$ volts verifies the wave nature of electrons by diffraction of the beam.

Read More

Magnetic field at the center of circular loop

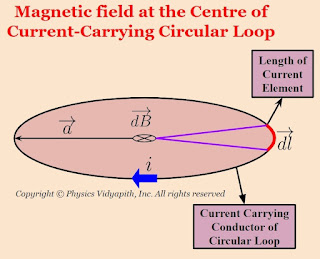
Mathematical Analysis of magnetic field at the center of circular loop:

Let us consider, a current-carrying circular loop of radius $a$ in which $i$ current is flowing. Now take a small length of a current element $dl$ so magnetic field at the center of a circular loop due to the length of current element $dl$. According to Biot-Savart Law:

$dB=\frac{\mu_{\circ}}{4 \pi} \frac{i .dl .sin\: \theta}{a^{2}} $

Here $\theta$ is the angle between length of current element $\left( \overrightarrow{dl} \right)$ and radius $\left( \overrightarrow{a} \right)$. These are perpendicular to each other i.e. $\theta = 90^{\circ}$

$dB=\frac{\mu_{\circ}}{4 \pi} \frac{i .dl .sin\: 90^{\circ}}{a^{2}} $

$dB=\frac{\mu_{\circ}}{4 \pi} \frac{i .dl }{a^{2}} \qquad \left(1 \right)$

The magnetic field at the center due to a complete circular loop

$B=\int dB \qquad \left(2 \right)$

From equation $(1)$ and equation $(2)$

$B=\int \frac{\mu_{\circ}}{4 \pi} \frac{i .dl}{a^{2}}$

$B=\frac{\mu_{\circ}}{4 \pi} \frac{i}{a^{2}} \int dl $

For complete loop $\int dl = 2 \pi a$, So from above equation that can be written as

$B=\frac{\mu_{\circ}}{4 \pi} \frac{i}{a^{2}} \left( 2 \pi a \right) $

$B=\frac{\mu_{\circ} i}{2 a}$

This is an equation of the magnetic field at the center of the circular loop.

Read More

Ampere's Circuital Law and its Modification

Ampere's Circuital Law Statement: When the current flows in any infinite long straight conductor then the line integration of the magnetic field around the current-carrying conductor is always equal to the $\mu_{0}$ times of the current.

$\int \overrightarrow{B}. \overrightarrow{dl} = \mu_{\circ} i$

Derivation of Ampere's Circuital Law: Let us consider, An infinite long straight conductor in which $i$ current is flowing, then the magnetic field at distance $a$ around the straight current carrying conductor

$B=\frac{\mu_{\circ}}{2 \pi} \frac{i}{a}$

Now the line integral of the magnetic field $B$ in a closed loop is

$\oint \overrightarrow{B}. \overrightarrow{dl} = \oint \frac{\mu_{\circ}}{2 \pi} \frac{i}{a} dl$

$\oint \overrightarrow{B}. \overrightarrow{dl} = \frac{\mu_{\circ}}{2 \pi} \frac{i}{a} \oint dl$

$\oint \overrightarrow{B}. \overrightarrow{dl} = \frac{\mu_{\circ}}{2 \pi} \frac{i}{a} \left(2 \pi a \right) \qquad \left( \because \oint dl= 2 \pi a \right)$

$\oint \overrightarrow{B}. \overrightarrow{dl} = \mu_{\circ}i$

Modified Ampere's circuital law: When the capacitor is placed in between the conductors then a current flows in the capacitor which is known as displacement current $\left(i_{d} \right)$. So modified Ampere's circuital law:

$\oint \overrightarrow{B}. \overrightarrow{dl} = \mu_{\circ} \left( i + i_{d} \right)$

Read More

Preparation of nanostructured particles by sol-gel method

Introduction:

The advent of the sol-gel process occurred in the year $1921$. In the $1960s$, its development was given due to the need for new synthesis methods in the nuclear industry.

The sol-gel method is a widely used wet chemical technique to fabricate nanostructured materials. This technique is used to prepare nanoparticles of ceramics, glassy, and composite materials at relatively low temperatures based on wet chemical processing. It involves the conversion of a precursor solution (i.e. sol) into a solid three-dimension network (i.e. gel) through hydrolysis and condensation reactions of precursor compounds. There are following steps are given below to fabricate nanostructured material through the sol-gel method.

1. Precursor Compound Selection: The first step involves selecting the appropriate precursor compounds, usually metal alkoxides or inorganic salts, that will form the desired material upon hydrolysis and condensation. These precursors should be soluble in a solvent i.e. metal salt in water or metal alkoxide in an organic solvent like alcohol.

2. Hydrolysis (Formation of Sol): It involves the conversion of a homogeneous solution of the precursor into a colloidal solution (i.e. The colloidal particles of precursor stably disperse in a solvent is called a Sol or colloidal solution.). In the hydrolysis process, Alcohol like ethanol or isopropanol is used as a solvent. Water or an acidic/basic catalyst is then introduced to initiate hydrolysis of the precursor molecules. This results in the breaking of metal-oxygen bonds in the precursor, generating metal hydroxide or oxide species. The reactions are given below.

$M-OR + H_{2}O \rightarrow M-OH +R-OH$

Where

$M=OR \rightarrow$ Metal Alkoxide
$M-OH \rightarrow$ Metal Hydroxide
$M \rightarrow Si, Ti,\: Zn,\: Al,\: Sn \:\: etc $

3. Condensation (Formation of Gel): The colloidal solution is kept for aging. During aging, the various condensation chemical reaction (i.e. polymerization) between two metal hydroxyl species leads to $M-O-M$ bonds with the release of $h_{2}O / R-OH$. This condensation process continues till finally results in a gel interconnected, rigid, and porous inorganic networks covered completely with the liquid phase. This transformation is known as a sol-gel transition.

$M-OR + HO=M \rightarrow M-O-M +R-OH$

4. Gelation: As the condensation reactions continue, the sol transforms into a three-dimensional network with a continuous solid phase interspersed with a liquid phase. This semi-solid network is known as a gel. The gelation process can be controlled by adjusting factors like precursor concentration, solvent composition, pH, and temperature.

5. Drying: Once the gel has formed, the excess solvent is removed through a drying process. The drying can be done by various techniques such as evaporation, supercritical drying, or freeze-drying. Careful drying is essential to prevent cracking or collapse of the gel structure.

6. Xerogel: If the solvent is dehydrated under ambient conditions (i.e. Removal of $R-OH$ groups). Xerogel is produced.

7. Calcination: In many cases, the dried gel must undergo a thermal treatment called calcination. This involves heating the gel at elevated temperatures to remove any remaining organic components and to induce further crystallization and growth of the desired material. The final crystalline structure and properties of the nanostructured material are developed during this step.

8. Characterization: The resulting nanostructured material is then characterized using various techniques like X-ray diffraction (XRD), scanning electron microscopy (SEM), transmission electron microscopy (TEM), and spectroscopy to analyze its composition, structure, particle size, and morphology.

Advantages of the Sol-gel process:

Versatile: Sol-gel method provides better control over the structure of particles, size of particles, and porosity. There is a possibility of incorporating nanoparticles and organic materials into sol-gel derived oxides.

Extended composition ranges: The sol-gel method allows the preparation of any oxide composition as well as also some non-oxides and the production of new hybrid organic-inorganic materials, which do not exist naturally.

Better Homogeneity: Due to mixing at the molecular level; high purity

Less energy consumption: There is no requirement for the melting temperature since the three-dimensional network structure can be achieved at relatively low temperatures.

Coating and thin films, monoliths, composites, porous membranes, powders, and fibers.

No need for special or expensive equipment.

Disadvantages of Sol-Gel:

Cost of precursors

Shrinkage of a wet gel upon drying, which often leads to fracture due to the generation of large capillary stresses and consequently, makes difficult the attainment of large monolithic pieces

Preferential precipitation of a particular oxide during the formation of colloidal solution i.e. Sol (in multicomponent glasses) due to the different reactivity of the alkoxide precursors

Difficult to avoid residual porosity and $OH$ groups.

Application of Sol-Gel:

Protective Coating

Thin films and fibers

Opto-mechanicalspan

Nanoscale powders

Medicine

Read More