Davisson and Germer's Experiment on Electron Diffraction:
Davisson and Germer's experiment verifies the wave nature of electrons with the help of diffraction of the electron beam as wave nature exhibits the diffraction phenomenon.
Principle: The principle of Davisson and Germer's experiment is based on the diffraction phenomenon of the electron beam by crystal and it verifies the de-Broglie relation.
Theoretical Formula: If a narrow beam of electrons is accelerated by a potential difference $V$ volts, the kinetic energy $K$ acquired by each electron in the beam is given by
$K=eV \qquad(1)$
Where $e$ is the charge of an electron
The de-Broglie wavelength is given by
$\lambda = \frac{h}{\sqrt {2m_{\circ} K \left( 1+ \frac{E_{K}}{2m_{\circ}c^{2}} \right)}}$
If $E_{K} \lt \lt 2m_{\circ}c^{2}$, then the term $\frac{E_{K}}{2m_{\circ}c^{2}}$ will be negligible. So above equation can be written as
$\lambda = \frac{h}{\sqrt {2m_{\circ} K}} \qquad(2)$
Now subtitute the value of $K$ from equation $(1)$ to equation $(2)$ then equation $(2)$ can be written as
$\lambda = \frac{h}{\sqrt {2m_{\circ} eV}}$
Now substitute the numerical value of $h$, $m_{\circ}$ and $e$, we get
$\lambda = \frac{6.63 \times 10^{-34}}{2 \times 9.11 \times 10^{-31} \times 1.6 \times 10^{-19} \times V}$
$\lambda = \frac{6.63 \times 10^{-9}}{29.15\times V}$
$\lambda = \frac{12.28}{\sqrt{V}}A^{\circ} \qquad(3)$
This is the theoretical value of $\lambda$ for the known potential difference in volts.
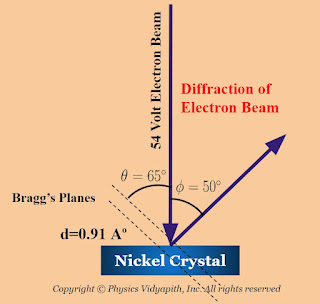
The calculation shows that the wavelength of the waves associated with the beam of electrons is of the same order as that of X-rays. Therefore, if such a beam of electrons is reflected from a crystal, the reflected beam will show the same diffraction and interference phenomena as for X-rays of the same wavelength. This consideration was the basis of Davisson and Germer's experiments. In one set of experiments, the [111] face of the nickel crystal was arranged perpendicular to the incident beam of electrons.
Apparatus:
There are the following apparatus is used
in the Davisson and Germer experiment.
1. Electron gun
2. Target
3. Electron detector or Faraday's cylinder
4. Galvanometer
1. Electron gun: The electron gun is a device that is used to produce a highly accelerated and collimated electron beam by applying high potential.
2. Target: It is a single large metal crystal i.e. nickel used as a target. In crystal, the atoms are arranged in regular lattice i.e. [111] so that the surface lattice of the crystal acts as a diffraction grating and the electrons get diffracted by the crystal in different directions. The electron beam is incident normal to the nickel crystal. The crystal can be rotated about an axis perpendicular to the incident beam so that various azimuthal angles could be used.
3. Electron detector or Faraday's cylinder: It is used to detect or measure the intensity of diffracted beams of electrons. It can be moved along a circular scale $S$. This electron detector is connected to a Galvanometer.
4. Galvanometer: The galvanometer is a device that is used to measure the very small amount of current following in the circuit.
The whole apparatus is completely enclosed and highly evacuated.
Working: When a low potential is applied to the electron gun so that a beam of slow electrons emerges from the gun and falls normally on the surface of the crystal. To collect the diffracted electrons, the Faraday cylinder is moved to various positions on the scale $S$ and the corresponding galvanometer measures the current at each position through an electron detector. The observation is repeated for electrons accelerated through different potentials. The current which is a measure of the intensity of the diffracted electron beam, is plotted against the diffracting angle $\phi$ for each accelerating potential as shown in the figure below
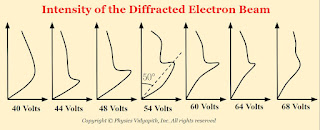
It is observed in the curves that at the voltage of $40$ volts, a smooth curve is obtained and a bump begins to appear in the curve for $44$ volts. As the potential difference is further increased, the bump starts shifting upward and becomes most prominent in the curve for $54$ volts at $\phi = 50 ^{\circ}$. Beyond $54$ volts the bump gradually diminishes and becomes insignificant at $68$ volts. The pronounced current peak at $54$ volts and at $50^{\circ}$ provided an evidence that electrons were diffracted by the target and verifies the existence of electron wave nature.
Calculation of Wavelength:
Theoretical Calculation:
The wavelength of electron at $54$ volts can be find by de- Broglie formula as shown in the equation $(3)$
$\lambda= \frac{12.28}{\sqrt{54}}\: A^{\circ}$
$\lambda= 1.671 \: A^{\circ} $
Experimental Calculation:
Experimental calculation is done by Bragg's diffraction equation
$n \lambda = 2d \: sin\theta$
Where
$d \rightarrow$ interplanar Spacing
$n \rightarrow$ Order of plane
For nickel crystal :
$n=1$
$d=0.91 A^{\circ}$
In the experiment, the diffracted electron beam appearing at $\phi=50 ^{\circ}$ aries from wave-like diffraction from the family of Bragg's planes.
The corresponding angle of incidence relative to the family of Bragg's planes is
$\theta = \frac{180 - \phi}{2}$
$\theta = \frac{180 - 50}{2}$
$\theta= 65^{\circ}$
Now apply these values to Bragg's equation as written above
$\lambda = 2 \times 0.91 \times sin 65^{\circ} $
$\lambda = 2 \times 0.91 \times 0.906 $
$\lambda = 1.65 A^{\circ} $
The theoretical and experimental value at $54$ volts verifies the wave nature of electrons by diffraction of the beam.